Perhaps surprisingly, that's actually good enough since the sum of the prime reciprocals also diverges. However, I'm not letting you just assume that, and proving it is harder than the original problem.
I can confirm that your intuition for divergence is correct.
This is related to the the May 16 post, but takes only the prime indexed terms. Does it still diverge?
Hint
Transform the product into a sum
Hint
The harmonic series 1 + 1/2 + 1/3 + ... 1/n +... diverges
At least 2 people I went to college with have bios. One became documentary filmmaker. I thought she was pleasant enough as a dormmate, but I don't think the administration liked her much. She's a bit nutty, but seems to have found her niche. The other is missing and presumed dead. He had a claim to notability as an academic before disappearing. He's not someone who'd do well as a missing person. Wikipedia won't say it without a reliable source identifying the corpse, but he's dead and has been since the day wandered off.
I thought a third guy had a good chance at an article, but it's just a redirect to the rodent he named himself after.
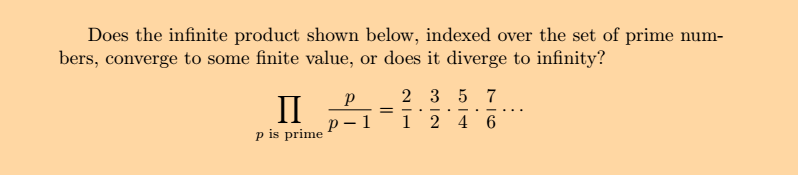
Solution
What I like about this solution is that it doubles as a proof that there are infinitely many prime numbers. It's not circular either -- it uses the number theoretic fact that every integer has a prime factorization, but nothing deeper. If only finitely many primes were required for that, ... well then of course every finite product converges.