this post was submitted on 10 May 2024
17 points (94.7% liked)
Daily Maths Challenges
216 readers
1 users here now
Share your cool maths problems.
Complete a challenge:
- Post your solution in comments, if it is exactly the same as OP's solution, let us know.
- Have fun.
Post a challenge:
- Doesn't have to be original, as long as it is not a duplicate.
- Challenges not riddles, if the post is longer than 3 paragraphs, reconsider yourself.
- Optionally include solution in comments, let it be clear this is not a homework help forums.
- Tag [unsolved] if you don't have a solution yet.
- Please include images, if your question includes complex symbols, attach a render of the maths.
Feel free to contribute to a series by DMing the OP, or start your own challenge series.
founded 11 months ago
MODERATORS
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments

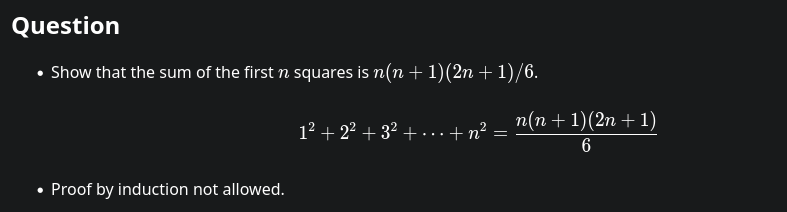
solution
Used some Minecraft for visualization on this one. Assumes you already know how to sum constants and sequential integers.When summing the odd integers 1 + 3 + 5 + ...,, they sum to the squares: The first n odd integers sum to n^2. This can be seen with a pretty simple construction, shown here - each additional layer, completing the next size square, has two more blocks than the previous one - they're the odd integers summed in sequence.
You can build a vaguely similar construction for the sum of squares - one quarter of a stepped pyramid, seen here. Seen from above in this view, it has the same structure as the sum of odds square on a per-layer basis. So each layer of the pyramid has an odd number of columns in it, in sequence, from 1 column for the highest layer to (2n-1) columns (the nth odd number) for the bottom layer. Each layer decreases in height by 1. So we can say that the 4th layer from the top, for example, has 7 columns in it (4th odd number), with n-3 blocks in each column (n in first layer, n-1 in second, n-2 in third, n-3 in fourth). So the columns in this layer have 7(n-3) blocks contained within them, total.
Going by this, we can write a sum to count the blocks.
Σ (i = 1 to n) n^2 = Σ (i = 1 to n) (2i-1) * (n-i+1)
The idea is we're counting up the blocks in all the columns that correspond to each distinct height. (2i-1) is giving us the i-th odd number, for the number of distinct columns at each level, while n-i+1 then gives us the number of blocks in each of those columns - and so their product counts the total blocks in all of those columns. All sums are from i = 1 to n, and that's omitted for less clutter. From here:
Σi^2 = Σ(2i-1) * (n-i+1)
Σi^2 = Σ(2in - 2i^2 + 3i - n - 1) → Expand, combine like terms
Σi^2 = (2n+3)Σi - 2Σi^2 - (n+1)Σ1 → Group by power of i, factor out constants
3Σi^2 = (2n+3)Σi - (n+1)Σ1 → Add 2Σi^2 to both sides
3Σi^2 = (2n+3)n(n+1)/2 - n(n+1) → Evaluate sums on right
3Σi^2 = ((2n+3)n(n+1) - 2n(n+1)) / 2 → Combine right to one fraction
3Σi^2 = n(n+1)(2n+1) / 2 → Factor n(n+1) from numerator, simplify
Σi^2 = n(n+1)(2n+1) / 6 → Divide both sides by 3, QED
Would like to mention I didn't view the hint, since I now see the hint mentions both constructions and Minecraft - the solution actually came to me before making it in Minecraft, but my attempts to explain it without images were pretty inadequate.
A second setup I also see, using the same stepped pyramid, is: Σ (i = 1 to n) (Σ (k = n - i + 1 to n) k). This one is summing up cross-sections of the pyramid perpendicular to the ground, from the light side of the pyramid to the heavy side. When i = n it's a full triangular cross-section (with 1 + 2 + 3 + ... + n blocks), but as you move across the pyramid, the top of the triangle (the side that starts at 1 block) gets cut off more and more - so when i = 1 it's just the bottom layer, with n blocks in it. This setup does also lead to a valid proof for the formula for Σi^2, if you work it out.
i took the second setup cuz thats what i saw when thinking of the problem, i'll read the first approach later
I did see a third setup where you take Σ (i = 1 to n) (Σ (k = 1 to i) 2k-1), which is a valid setup where you count all the blocks on the top of their column, then remove those and count the new top blocks, etc - but it ended up not leading to a proof of the formula because as it turns out, there are always i^2 (from i = n to 1) blocks on the top layer. So it's just a worse way of doing Σi^2 and we get a 0 = 0 situation.