this post was submitted on 12 Feb 2025
265 points (98.5% liked)
Map Enthusiasts
3794 readers
1 users here now
For the map enthused!
Rules:
-
post relevant content: interesting, informative, and/or pretty maps
-
be nice
founded 2 years ago
MODERATORS
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments
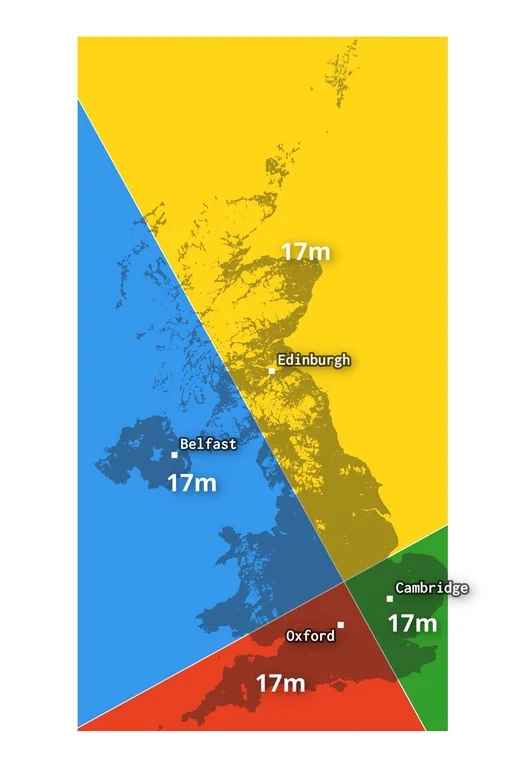
I can't find it now but I seem to remember a proof that a collection of points could be divided in half by a straight line, first by assuming that no three points were collinear, then by picking any point on the plane, drawing a straight line through it, then rotating it around that point until you could get half the points on each side. The implication of this though is that you could pick someone in Aberdeen, which the above map would seem to suggest isn't possible.
The location of the second line would have to be determined by sliding it along the first, rather than rotation, so it could end up resting on 0, 1 or 2 points. Either way you're probably close enough. The proof, for or against, is outside my mathematical ability.
In that description, the two lines wouldn't necessarily be perpendicular.
https://en.wikipedia.org/wiki/Ham_sandwich_theorem
You can bisect the population with a line at any angle or passing through any given point. Given any one bisecting line, you can find another line that splits the map into four equal quadrants, but the lines wouldn't necessarily be perpendicular.
I'll see if I can come up with a proof for a perpendicular quad-section, and reply to the comment above.
Edit: I can't. Not simple enough for me to find at the moment!