Science Memes

11189 readers

2476 users here now

Welcome to c/science_memes @ Mander.xyz!

A place for majestic STEMLORD peacocking, as well as memes about the realities of working in a lab.

Rules

Don't throw mud. Behave like an intellectual and remember the human.
Keep it rooted (on topic).
No spam.
Infographics welcome, get schooled.

This is a science community. We use the Dawkins definition of meme.

Research Committee

[email protected]

Other Mander Communities

Science and Research

Biology and Life Sciences

Physical Sciences

Humanities and Social Sciences

Practical and Applied Sciences

Memes

Miscellaneous

founded 2 years ago

MODERATORS

[email protected]

976

Academic writing (mander.xyz)

submitted 2 months ago by [email protected] to c/[email protected]

105 comments fedilink hide all child comments

you are viewing a single comment's thread
view the rest of the comments

[–] [email protected] 16 points 2 months ago (1 children)

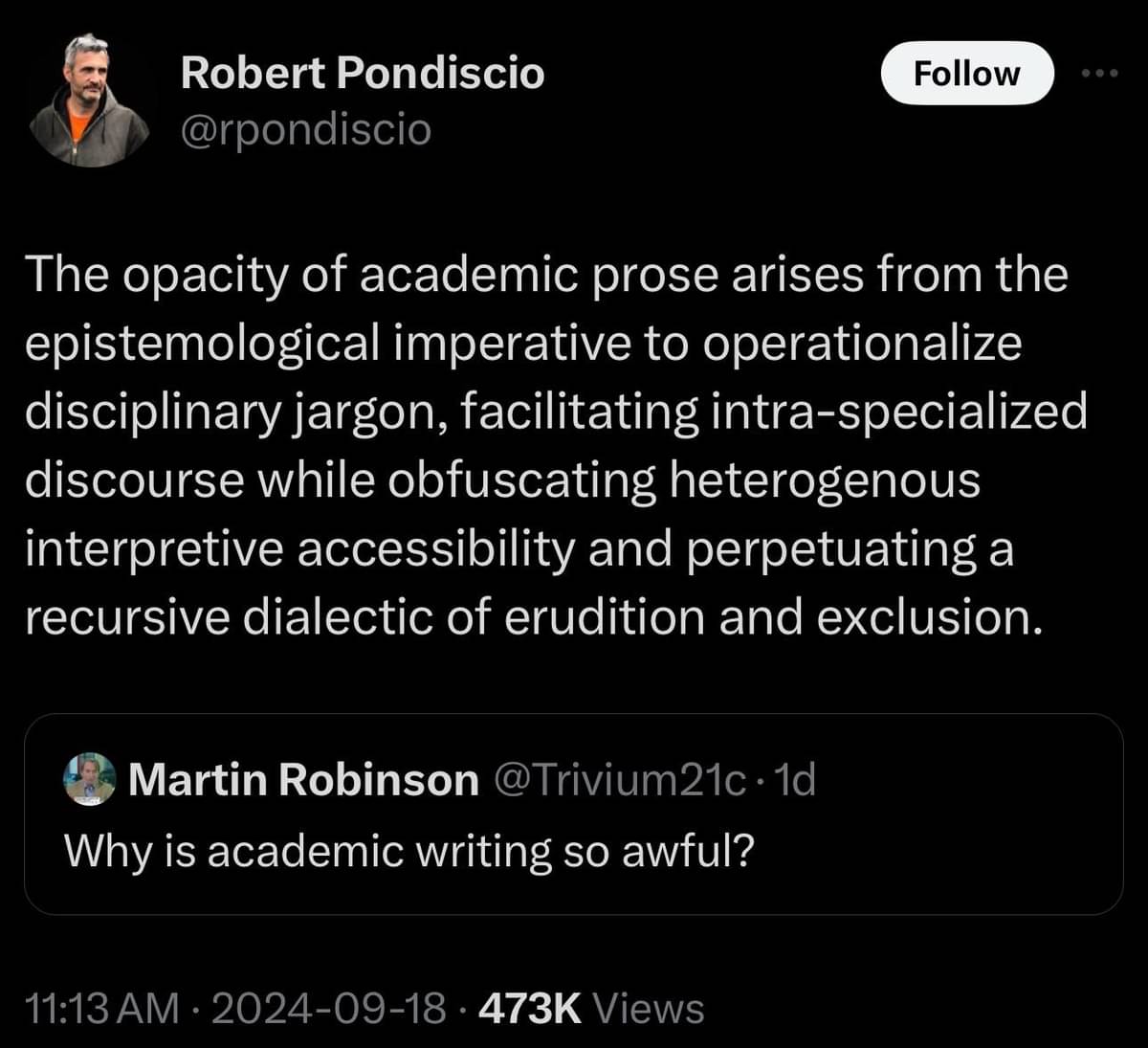
Gotta love Dirichlet boundary conditions (the function has to have this value), Neumann boundary conditions (the derivative has to have this value) and Cauchy boundary conditions (both).

On the other hand, there's a bunch of things that are so abstract that it's difficult to give them a descriptive name, like rings, magmas and weasels

[–] [email protected] 2 points 2 months ago

Oh i would say "ring" is in fact quite a descriptive term.

Apparently, in older german, "ringen" meant "to make progress of some sort/to fight for something". And a ring has two functions: addition and multiplication. These are the foundational functions that you can use to construct polynomials, which are very important functions. You could look at functions as a machine where you put something in and get something out.

In other words, you put something into a function, the function internally "makes some progress", and spits out a result. That is exactly what you can do with a "ring".

So it kinda makes sense, I guess.